Sir Harold Marshall died a few months back and was farewelled in his beloved Mount Albert Methodist Church. It is a congregation of which Sir Harold had been a member for most of his life and, as you might expect, his funeral was a poignant farewell from family, friends and members of the church. A constant theme in their addresses was the remarkable breadth of his interests. He was variously described as a poet, an architect, a watercolourist, a sailor, a horticulturist, a mystic and, of course, an acoustician.

We are most familiar with him as an acoustician, not the least from the recent release of Rick Harvie and Jane Mahoney’s documentary Maurice and I. The film features Sir Harold slowly climbing the stair to the upper level of the recently repaired Christchurch Town Hall and opening the door to exclaim that it was “as if it were new”. The moment was redolent with moving memories of the professional relationships that had begot that hall and, especially, of the three key protagonists, Maurice Mahoney, Sir Miles Warren and Sir Harold, all no longer with us. For those of us from Christchurch, it brought back, too, the transformational power of that great civic room in the city. Within its robust hide, cast from shingle off the surrounding plains, we were welcomed with Pat Hanly’s spectacular portal mural to a wonderful elliptical space that offered us everything from Leonard Bernstein to Carlos Santana.

For all of Sir Harold’s success at balancing audience proximity with clarity and richness of sound, it was his description as a mystic that I found most interesting. His lifelong membership of the church was unequivocal, but his identification as a mystic bespoke an investigation beyond the constraints of doctrinal teachings. I had a brief insight to this enquiry in discussions about the employment of the Fibonacci sequence to determine the configuration of a back wall of a performance space I was working on. I understood the sequence as a device for generating a geometry that was both ordered and rational, yet complex in its outcome, but I suspect any greater meaning of the sequence was lost on me then.

You will know the sequence as a run of numbers in which each is the sum of its preceding two numbers: thus 1, 1, 2, 3, 5, 8, etc. Closer investigation reveals that each number divided by its predecessor approximates the golden mean, the larger the numbers, the closer to the golden mean the product becomes. A seminal moment in my engagement with numbers had occurred early in a pure mathematics paper at Ilam when a branch of the subject concerning imaginary numbers made its appearance. I beat a hasty retreat to a more humanist line of enquiry at the old ‘town site’ campus, and to the beach. Turning away so completely from that new science campus in the suburbs may have been a mistake. While the liberal arts inform the big issues of architecture, they do little to assist us dealing with the complexities of Newtonian laws of nature that are ever with us. Thank god, the damned square root of minus one has not made a reappearance in 50 or so years of architectural practice.

As one increasingly works through the hand, and mouse, of those in the studio rather than wielding the tools, one has to be increasingly explicit about why it is that, for example, the division of mullions within a façade need be this rather than that. I have previously had to placate an irate recent graduate who, frustrated by my relentless critique, threw down his pencil in high dudgeon and demanded I ‘draw the damn elevation’ myself. I have, in response, come rather late to an understanding that, perhaps, the tentative armature of beliefs and hunches around which I construct projects is somewhat wanting. I might need to have a greater regard for the precision and clarity of theory and numbers to avoid the turmoil of disgruntled collaborators trying to discern purpose within my crude sketches.

I have an instinctive dislike of the prescriptive, though, and am pretty certain I won’t be sorting out my next elevation according to one or another Gaussian number series or the imperatives of the golden mean, though its ratios lurk ever present in my work. The pervasive nature and value of a structured approach to data was, however, underlined recently, albeit in a separate but not unrelated discipline.

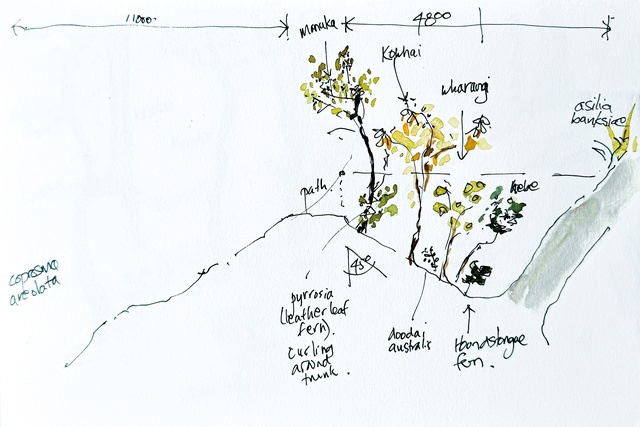
The studio headed north with landscape architect Philip Smith to become a little better acquainted with the flora of the Northland bays. This was achieved through the tabulation of transepts taken through a variety of coastal conditions. We were in groups, each tackling a different topography: the rocky outcrop above high water, a roadside drainage ditch, the ridge-top walking track to the beach, and so on.

The transept is an organising schema for illustrating data along a cross-section that some of us were familiar with from the work of new urbanists Duany Plater-Zyberk. Rather than revealing field, suburb and downtown as they did, Smith had us focus on traversing only a few metres of terrain. His descriptions of our hard-fought-for samples of grasses, branches and leaves uncovered a world of symbiotic interaction: a potent reminder of the complexity of the world immediately around us.

In each team, members were induced to clamber up and down slopes with tape and string line in hand in order to survey the plants along the line while others recorded their discoveries. Later in the evening, we made models of our particular terrain and presented our findings. As part of Smith’s judging of our barely suppressed competitive enthusiasms, we were treated to an erudite explanation of the plants, their Latin, common and Māori names, habitats, reliance on fauna for propagation and uses in traditional Māori life, and the interaction between species.

The work we architects do is circumscribed by physics: the shaping of Euclidean geometry to withstand the Newtonian forces of gravity and the clandestine assaults of earthquake and storm. For all that, it is at some remove from the symbiotic processes Smith described. His description of the impact that eons of deposited guano are likely to have had on the health of Northland pōhutukawa, and their possible decline as bird numbers and deposited guano have dropped, was a speculation that provoked consideration of inexorable forces moving to a time frame well beyond that of our daily labours.

The rate of change of that small bit of Northland ecology brought us to a discussion of Tutira and the deeply layered 60-year study of a Hawke’s Bay sheep farm by William Herbert Guthrie-Smith from the late 19th century. It is an intense observational study of geomorphic and ecological processes that shaped the land worked by the self-described ‘not altogether idiotic sheep-farmer’. His thoughtful speculations on the world he inhabited reveal, as did Sir Harold’s relentless enquiry, the enormous return to be gained from the empirical study of the world around us, be it leaf, rock, pile of guano or sequence of numbers.